Definitions
On notation: for an analytic function \(f\) expanded as a power series about \(a\), write \(v_a(f)\) as the \(a{\hbox{-}}\)adic valuation of \(f\): expanding \(f(z) = \sum_{k\in {\mathbb{Z}}} a_k (z-a)^k\) about \(a\), define \(v_a(f) = n\) iff \(a_n\neq 0\) but \(a_{\leq n} = 0\). In other words, \(v_a\) is the lowest power of \((z-a)\) occurring in a Laurent expansion of \(f\) about \(a\).
A point \(z_0\) is an isolated singularity if \(f(z_0)\) is undefined but \(f(z)\) is defined in a punctured neighborhood \(D(z_0)\setminus\left\{{z_0}\right\}\) of \(z_0\).
There is a classification of isolated singularities:
- Removable singularities, so \(v_a(f) \in [0, \infty)\)
- Poles, so \(v_a(f) \in (-\infty, 0)\)
- Essential singularities, so \(v_a(f) = -\infty\), e.g. \(\sin(z^{-1})\) at \(z=0\).
Not all singularities are isolated, and thus don’t fall into this classification. One may also have branch singularities, e.g. \(\operatorname{Log}(z)\) at \(z=0\). \(f(z) \coloneqq z^{1\over 2}\) has a singularity at zero that does not fall under this classification – the point \(z=0\) is a branch singularity and \(f\) admits no Laurent expansion around \(z=0\). A similar example: \(\qty{z(z-1)}^{1\over 2}\) has two branch singularities at \(z=0, 1\).
Singularities can be classified by Laurent expansions \(f(z) = \sum_{k\in {\mathbb{Z}}} c_k z^k\):
- Essential singularity: infinitely many negative terms.
- Pole of order \(N\): truncated at \(k = -N\), so \(c_{N-\ell} = 0\) for all \(\ell\).
- Removable singularity: truncated at \(k=0\), so \(c_{\leq -1} = 0\).
Isolated singularities can also be classified by their limiting behavior near the singularity:
- \(\lim_{z\to z_0} f(z) < \infty\): removable (equivalently: bounded in a neighborhood).
- \(\lim_{z\to z_0} f(z) = \infty\): pole.
- \(\lim_{z\to z_0} f(z)\) does not exist: essential.
For any \(f\) holomorphic on an unbounded region, we say \(z=\infty\) is a singularity (of any of the above types) of \(f\) if \(g(z) \coloneqq f(1/z)\) has a corresponding singularity at \(z=0\).
Removable
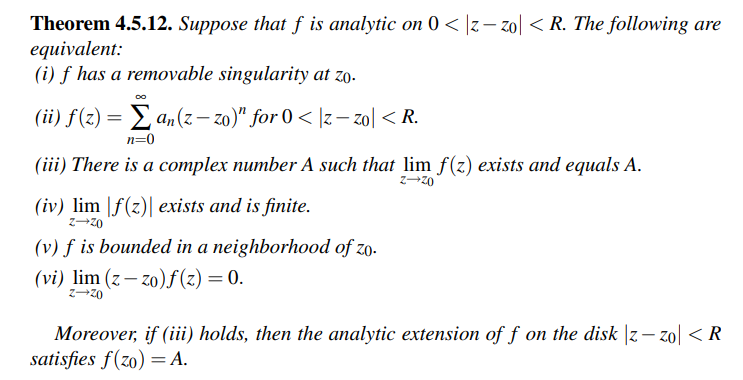
If \(z_0\) is a singularity of \(f\). then \(z_0\) is a removable singularity iff there exists a holomorphic function \(g\) such that \(f(z) = g(z)\) in a punctured neighborhood of \(z_0\). Equivalently, \begin{align*} \lim_{z\to z_0}(z-z_0) f(z) = 0 .\end{align*} Equivalently, \(f\) is bounded on a neighborhood of \(z_0\). Equivalently, \(v_{z_0}(f) \geq 0\)
- \(f(z) \coloneqq\sin(z)/z\) has a removable singularity at \(z=0\), and one can redefine \(f(0) \coloneqq 1\).
- If \(f(z) = p(z)/q(z)\) with \(q(z_0)=0\) and \(p(z_0)=0\), then \(z_0\) is removable with \(f(z_0)\coloneqq p'(z_0)/q'(z_0)\).
Suppose \(f\) is holomorphic on \(\Omega\setminus\left\{{z_0}\right\}\). TFAE:
- \(z_0\) is a pole of order \(0\).
- \(z_0\) is a removable singularity of \(f\).
- There exists some neighborhood of \(z_0\) on which \(f\) is bounded.
- \((z-a)f(z) \overset{z\to z_0}\longrightarrow 0\)
- \(f\) admits a holomorphic extension \(F\) to all of \(\Omega\)
- \(f\) admits a continuous extension \(F\) to all of \(\Omega\).
- \(f\) admits a Laurent expansion about \(z_0\) with vanishing principal part, i.e. \(f(z) = \sum_{k\geq 0}c_k (z-z_0)^k\).
Take \(\gamma\) to be a circle centered at \(z_0\) and use \begin{align*} f(z) \coloneqq\int_\gamma { f(\xi) \over \xi - z} \,dx .\end{align*} This is valid for \(z\neq z_0\), but the right-hand side is analytic. (?)



A singularity of a holomorphic function is removable if and only if the function is bounded in some punctured neighborhood of the singular point.
Essential
A singularity \(z_0\) is essential iff it is neither removable nor a pole. Equivalently, a Laurent series expansion about \(z_0\) has a principal part with infinitely many terms.
\(f(z) \coloneqq e^{1/z}\) has an essential singularity at \(z=0\), since we can expand and pick up infinitely many negative terms: \begin{align*} e^{1/z} = 1 + {1\over z} + {1\over 2! z^2} + \cdots .\end{align*} In fact there exists a neighborhood of zero such that \(f(U) = {\mathbb{C}}\setminus\left\{{0}\right\}\). Similarly \(g(z) \coloneqq\sin\qty{1\over z}\) has an essential singularity at \(z=0\), and there is a neighborhood \(V\) of zero such that \(g(V) = {\mathbb{C}}\).
The singularities of a rational function are always isolated, since there are finitely many zeros of any polynomial. The function \(F(z) \coloneqq\operatorname{Log}(z)\) has a singularity at \(z=0\) that is not isolated, since every neighborhood intersects the branch cut \((-\infty, 0) \times\left\{{ 0 }\right\}\), where \(F\) is not even defined. The function \(G(z) \coloneqq 1/\sin(\pi/z)\) has a non-isolated singularity at 0 and isolated singularities at \(1/n\) for all \(n\).
Zeros
A subset of \({\mathbb{R}}^n\) is closed and bounded iff it is sequentially compact. Equivalently, every bounded sequence has a convergent subsequence.
Why this is useful: every infinite subset of a disk has a limit point. So e.g. if \(f\) is holomorphic and has infinitely many zeros in \({\mathbb{D}}\), \(f\) is identically zero by the identity principle.
A zero of an analytic function on a domain \(\Omega\) is any \(z_0\) such that \(f(z_0)=0\), with no further conditions. If \(f\) is analytic and not identically zero on \(\Omega\) with \(f(z_0) = 0\), then there exists a neighborhood \(U\ni z_0\) and function \(g\) that is holomorphic and nonvanishing on \(U\) such that \begin{align*} f(z) = (z-z_0)^n g(z) .\end{align*} We refer to \(z_0\) as a zero of order \(n\). Equivalently, \(f^{(n-1)}(z_0)=0\) but \(f^{(n)}(z) \neq 0\), so the Laurent expansion has the form \(f(z) = \sum_{k\geq n} c_k (z-z_0)^k\) where \(c_n\neq 0\).
On terminology: if the order of \(z_0\) for \(f\) is \(n\), we say \(f\) vanishes to order \(n\).
Use that \(\Omega\) is connected to find some neighborhood \(U\) on which \(f\) is not identically zero. WLOG assume \(z_0=0\). Expand \(f\) as an honest power series at \(z_0\) to write \begin{align*} f(z) = \sum_{k\geq 0}c_k z^k = z^n\qty{c_n + c_{n+1}z + \cdots} \coloneqq z^n g(z) ,\end{align*} where \(a_n\) is the minimal nonvanishing coefficient. Since \(a_n\neq 0\), we have \(\lim_{z\to z_0} g(z) = a_n \neq 0\), so \(g\) is nonvanishing in some neighborhood of \(z_0\). Uniqueness follows from writing \begin{align*} z^n g(z) = z^m h(z) \implies g(z) = z^{m-n} h(z) ,\end{align*} assuming \(m>n\), but then taking \(z\to z_0 =0\) on the RHS yields \(g(z) = 0\), a contradiction.

If \(f:{\mathbb{C}}\to {\mathbb{C}}\) is holomorphic and not identically zero, then \(f\) has isolated zeros.
Suppose not, then pick a limit point \(z_0\) with \(f(z_0)=0\) with a sequence \(\left\{{z_k}\right\}\to z_0\) where \(f(z_k) = 0\) for all \(k\). Expand \(f\) in a Laurent series; since \(f\not\equiv 0\) there is a smallest nonzero coefficient \(c_m\): \begin{align*} f(z) = \sum_{k\geq m}c_k (z-z_0)^k = c_m(z-z_0)^m \cdot\qty{1 + \sum_{k\geq 1}c_k' (z-z_0)^k } \coloneqq c_m(z-z_0)^m \cdot(1 + g(z-z_0)) .\end{align*} Note \(g(z-z_0)\overset{z\to z_0}\longrightarrow 0\), and since \(z_k\to z_0\) we can find \(k\gg 1\) such that \(g(z_k - z_0) < {\varepsilon}\). In particular, for \(k\) large enough, \(1 < 1+g(z_k - z_0) 1 + {\varepsilon}\), but this contradicts \(f(z_k) = 0\): \begin{align*} 0 = f(z_k) = c_m(z_k - z_0)^m (1 + g(z_k - z_0)) \neq 0 .\end{align*}
\(\contradiction\)
If \(f,g\) are holomorphic and \(f=g\) on any set with a limit point, then \(f\equiv g\).
The proof follows from the fact that \(f-g\) is holomorphic and has nonisolated zeros.

Poles
Let \(f\) be a meromorphic function with an isolated singularity at \(z_0\). TFAE:
- \(z_0\) is a pole of \(f\) of order \(n\).
- \({\left\lvert {f(z)} \right\rvert}\overset{z\to z_0}\longrightarrow \infty\)
- \(z_0\) is a zero of order \(n\) of \(g(z) \coloneqq{1\over f(z)}\), and \(g\) is holomorphic in a neighborhood of \(z_0\)
- \(f(z) = (z-z_0)^{-n}h(z)\) where \(h\) is holomorphic in a punctured neighborhood of \(z_0\).
- \(f\) admits a Laurent expansion of the form \begin{align*} f(z) = \sum_{k\geq -n} c_k (z-z_0)^k, && c_{-n}\neq 0 .\end{align*}
Any pole admits a neighborhood where \(f\) is nonvanishing, and in fact bounded below.
In this case there exists a minimal \(n\) and a holomorphic \(h\) such that \begin{align*} f(z) = \qty{z-z_0}^{-n} h(z) .\end{align*} Such an \(n\) is the order of the pole. A pole of order 1 is said to be a simple pole.
Use that \(z_0\) is a zero of \(1/f\) to write \begin{align*} {1\over f(z) } = (z-z_0)^n g(z) ,\end{align*} for \(h\) holomorphic and nonvanishing in a neighborhood of \(z_0\). Taking reciprocals yields \begin{align*} f(z) = (z-z_0)^{-n} h(z) && \quad h(z) \coloneqq{1\over g(z)} .\end{align*}
Claim: if \(f\) has a pole of order \(m\) at \(z_0\), then \(g(z) \coloneqq f(z^2)\) has a pole of order \(2m\) at \(z_0\). WLOG assume \(z_0=0\). Note that this is clear by multiplying Laurent expansions about \(z_0\): \begin{align*} f(z) = \sum_{k\geq -m} c_k z^k \implies g(z) = \sum_{k\geq -m} c_k z^{2k} = {c_{-m} \over z^{2m}} + \cdots .\end{align*} Using the other characterization, write \(f(z) = z^{-m} h(z)\) with \(g\) holomorphic and nonzero in a neighborhood \(U\) of \(z_0\), so in particular \(h(0) \neq 0\). Then \(f(z^2) = z^{-2m} h(z^z)\) and \(h(z^2)\mathrel{\Big|}_{z=0} = h(0) \neq 0\).
If \(f\) has a pole of order \(n\) at \(z_0\), then there exist a holomorphic \(G\) in a neighborhood of \(z_0\) such that \begin{align*} f(z) = {a_{-n} \over (z-z_0)^n } + \cdots + {a_{-1} \over z-z_0} + G(z) \coloneqq P(z) + G(z) .\end{align*}
The term \(P(z)\) is referred to as the principal part of \(f\) at \(z_0\) consists of terms with negative degree, and the residue of \(f\) at \(z_0\) is the coefficient \(a_{-1}\).