Residues – Notes

remark:

On strategy: see https://www.damtp.cam.ac.uk/user/reh10/lectures/nst-mmii-chapter5.pdf

A quick shortcut (?) for the quotient rule: $\begin{align*} {\frac{\partial }{\partial z}\,} {p(z) \over q(z)} = {p'(z)\over q(z)} - {p(z)q'(z) \over q^2(z)} .\end{align*}$ Useful when taking $z\to z_0$ with $z_0$ a root of $p, p', q'$ .

remark:

Pedantic warning: $\mathop{\mathrm{Res}}_{z=p}(f)$ should really be $\mathop{\mathrm{Res}}_{z=p}(df)$ for $df = f(z) \,dz$ , since it’s only an invariant of the 1-form $df$ and not necessarily $f$ itself. We freely abuse notation!

remark:

Check: do you need residues at all?? You may be able to just compute an integral!

If the integrand is holomorphic throughout the region enclosed by $\gamma$ , $\int_\gamma f = 0$
If $f$ has a well-defined primitive $F$ on $\gamma$ , then $\begin{align*} \int_\gamma f = \int_\gamma F' = F(\gamma(1)) - F(\gamma(0)) = 0 .\end{align*}$
Use Cauchy’s theorem when applicable: $\begin{align*} \int_\gamma {f(z) \over (z-a)^n} = 2\pi i f^{(n-1)}(a) .\end{align*}$
Compute directly by parameterization: $\begin{align*} \int_\gamma f \,dz= \int_a^b f(z(t))\, z'(t) \,dt&& \text{for } z(t) \text{ a parameterization of } \gamma ,\end{align*}$
- Note: you can parameterize a circle around $z_0$ using $\begin{align*} z= z_0 + re^{i \theta } .\end{align*}$

Residue Formulas

theorem (The residue theorem):

Let $f$ be meromorphic on a region $\Omega$ with poles $\left\{{ { {z}_1, {z}_2, \cdots, {z}_{N}} }\right\}$ . Then for any $\gamma \in \Omega\setminus\left\{{ { {z}_1, {z}_2, \cdots, {z}_{N}} }\right\}$ , $\begin{align*} {1 \over 2\pi i } \int_\gamma f(z) \,dz= \sum_{j=1}^N n_\gamma(z_j) \mathop{\mathrm{Res}}_{z=z_j} f .\end{align*}$ If $\gamma$ is a toy contour with winding number 1 about each pole, then $\begin{align*} {1\over 2\pi i}\int_\gamma f\,dz= \sum_{j=1}^N \mathop{\mathrm{Res}}_{z=z_j}f .\end{align*}$

theorem (The residue formula):

If $f$ has a pole $z_0$ of order $n$ , then $\begin{align*} \mathop{\mathrm{Res}}_{z=z_0} f = \lim_{z\to z_0} {1 \over (n-1)!} \qty{{\frac{\partial }{\partial z}\,}}^{n-1} (z-z_0)^n f(z) .\end{align*}$

As a special case, if $z_0$ is a simple pole of $f$ , then $\begin{align*} \mathop{\mathrm{Res}}_{z=z_0}f = \lim_{z\to z_0} (z-z_0) f(z) .\end{align*}$

corollary (Residue formula: rational function formula for simple poles):

If additionally $f=g/h$ where $h(z_0) = 0$ and $h'(z_0)\neq 0$ , $\begin{align*} \mathop{\mathrm{Res}}_{z=z_0} {g(z) \over h(z)} = {g(z_0) \over h'(z_0)} .\end{align*}$

Note that if $f(z) = 1/h(z)$ and $z_0$ is a simple pole, this reduces to $\begin{align*} \mathop{\mathrm{Res}}_{z=z_0}{1\over h(z)} = {1\over h'(z_0)} .\end{align*}$

warnings:

Note that only the denominator gets differentiated, not the numerator! To remember this, just rederive the equation from L’Hopital’s rule and use the product rule on $(z-z_0)g(z)$ .

proof (Of derivative formula for simple poles):

Apply L’Hopital: $\begin{align*} (z-z_0) {g(z) \over h(z)} = {(z-z_0) g(z) \over h(z) } \overset{LH}{=} {g(z) + (z-z_0) g'(z) \over h'(z)} \overset{z\to z_0}\longrightarrow{g(z_0) \over h'(z_0)} .\end{align*}$

theorem (Residue formula: poles at infinity):

$\begin{align*} \mathop{\mathrm{Res}}_{z=\infty}f(z) = \mathop{\mathrm{Res}}_{z=0} g(z) && g(z) \coloneqq-{1 \over z^2}f\qty{1\over z} .\end{align*}$

Note on where this weird formula comes from: residues are associated not to function $f$ but to differential forms $f(z)\,dz$ , and inversion sends $f(z) \,dz\to f(1/z)d(1/z) = f(1/z)\cdot -{1\over z^2}\,dz$ . This residue can alternatively be calculated for $f$ by taking $\gamma$ a contour enclosing all singularities of $f$ and computing $\begin{align*} \mathop{\mathrm{Res}}_{z=\infty}f(z) = -{1\over 2\pi}\int_\gamma f(z) \,dz .\end{align*}$

theorem (Residue formula: fractional residues):

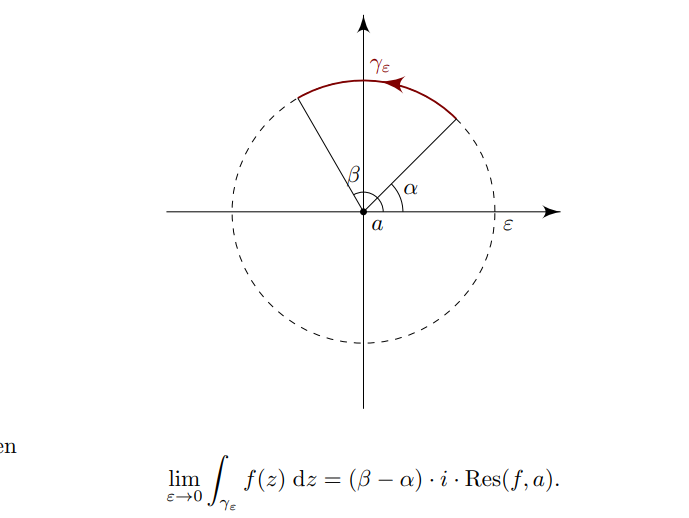
If $z_0$ is an order 1 pole of $f$ and $\gamma_{{\varepsilon}, \theta}$ is an arc of the circle $C_{\varepsilon}\coloneqq\left\{{ {\left\lvert {z-z_0} \right\rvert} = {\varepsilon}}\right\}$ subtending an angle of $\theta$ , then $\begin{align*} \lim_{{\varepsilon}\to 0} \int_{\gamma_{{\varepsilon}, \theta}} f(z) \,dz= i\theta \mathop{\mathrm{Res}}_{z = z_0}f(z) .\end{align*}$

figures/2021-12-22_05-13-02.png

proof (?):

figures/2021-12-22_05-13-27.png

Exercises

Avoiding Residue Formulas

exercise (Integrating $z^k$ around $S^1$ is the source of residue theory):

Show that $\begin{align*} \int_{S^1}z^k \,dz= \begin{cases} 2\pi i & k=-1 \\ 0 & \text{else}. \end{cases} ,\end{align*}$ and thus $\begin{align*} \int \sum_{k\geq -M} c_k z^k = \sum_{k\geq -M} \int c_k z^k = 2\pi i c_{-1} ,\end{align*}$ i.e. the integral picks out the $c_{-1}$ coefficient in a Laurent series expansion.

#complex/exercise/completed

solution:

$\begin{align*} \int_\gamma z^k \,dz= \int_0^{2\pi} e^{ik\theta} ie^{i\theta } \,d\theta= i\int_0^{2\pi} e^{i(k+1)\theta \,d\theta} = \begin{cases} 2\pi i & k=-1 \\ 0 & \text{else}. \end{cases} \end{align*}$

exercise (Primitive in the complement of a branch cut):

Compute the following integrals: $\begin{align*} \int_{{\left\lvert {z-1} \right\rvert} = 1} {1\over z^2-1} \,dz\\ \int_{{\left\lvert {z-0} \right\rvert} = 2} {1\over z^2-1} \,dz\\ .\end{align*}$ Compute the 2nd integral by finding a primitive.

#complex/exercise/completed

solution:

For the first integral: $\begin{align*} \int_\gamma{1\over z^2-1}\,dz &= {1\over 2}\int_\gamma {1\over z-1} + {1\over z+1}\,dz\\ &= {1\over 2}\int_\gamma {1\over z-1} \\ &= {1\over 2}\cdot 2\pi i \mathop{\mathrm{Res}}_{z=1}f(z) \\ &= {1\over 2}\cdot 2\pi i \cdot 1 \\ &= \pi i ,\end{align*}$ using that $f(z) = {1\over z-1}$ is already an expansion of $f$ about $z=1$ since it is a Laurent series in $(z-1)^k$ , so the residue is $1$ .

For the second integral: attempt to define a primitive $\begin{align*} F(z) \coloneqq{1\over 2}\log\qty{z-1\over z+1} \implies F'(z) = {1\over z^2-1} .\end{align*}$ If this primitive is well-defined on $\gamma$ , the integral will vanish because this is a closed curve. Choose the branch cut ${\mathbb{C}}\setminus(-\infty, 0]$ and define $g(z) = {z-1\over z+1}$ , so that $F(z) = {1\over 2}\log(g(z))$ . Then then $g(z)\in (-\infty, 0] \iff z\in [-1, 1]$ , and this is well-defined on $\gamma$ since $[-1, 1]$ does not intersect $\gamma$ .

Thus for $\gamma$ any parameterization of ${\left\lvert {z} \right\rvert} = 2$ , $\begin{align*} \int_{{\left\lvert {z} \right\rvert} = 2} f(z) \,dz = \int_{{\left\lvert {z} \right\rvert} = 2} F'(z)\,dz = F(\gamma(0)) - F(\gamma(1)) = 0 .\end{align*}$

exercise (Cauchy formula and $\sinh$):

Compute $\begin{align*} \int_{S^1} {2 \sinh(z) \over z^n}\,dz .\end{align*}$

#complex/exercise/completed

solution:

Write $f(z) = 2\sinh(z) = e^{z} - e^{-z}$ and apply the generalized Cauchy formula: $\begin{align*} f^{(n-1)}(0) &= {(n-1)! \over 2\pi i} \int_{S^1} {f(z) \over (z-0)^n}\,dz\\ \\ \implies \int_{S^1}{f(z)\over z^n}\,dz &= {2\pi i \over (n-1)!} f^{(n-1)}(0) \\ &= {2\pi i\over (n-1)!} 2\cdot \qty{e^z - (-1)^{n-1} e^z \over 2}\Big|_{z=0} \\ &= {2\pi i\over (n-1)!} 2\cdot \qty{1 + (-1)^{n} \over 2} \\ &= \begin{cases} {2\pi i \over (n-1)!} & n \text{ even } \\ 0 & n \text{ odd }. \end{cases} .\end{align*}$

exercise (Cauchy formula and principal parts):

Compute $\begin{align*} \int_\gamma {z^2+1 \over z(z^2 + 4)}\,dz \end{align*}$ for

A circle of radius $1$ ,
A circle of radius $2+{\varepsilon}$ ,

#complex/exercise/completed

solution:

For the smaller circle, use Cauchy’s formula $\begin{align*} \int_{{\left\lvert {z} \right\rvert} = 1} f(z) \,dz &= \int_{{\left\lvert {z} \right\rvert} = 1} { {z^2 + 1 \over z^2 + 4} \over z} \,dz\\ &= 2\pi i \qty{z^2 + 1 \over z^2 + 4} \Big|_{z=0} \\ &= {i\pi \over 2} .\end{align*}$ .

For the larger circle, break into principal parts. Write $\begin{align*} {z^2 + 1 \over z(z^2+4)} = {a\over z} + {b\over z+2i} + {c\over z-2i} ,\end{align*}$ and use PFD/cover-up method/finding residues:

$a = \qty{z^2+1\over z^2+4}\Big|_{z=0} = {1\over 4}$
$b= \qty{z^2 + 1 \over z(z-2i)}\Big|_{z=-2i} = {-4+1\over -2i(-4i)} = {3\over 8}$
$c = \qty{z^2 + 1 \over z(z+2i)}\Big|_{z=2i} = {-4+1 \over 2i(4i)} = {3\over 8}$

Thus $\begin{align*} \int_\gamma f(z) \,dz &= \int_\gamma \qty{ {1/4\over z} + {3/8\over z+2i} + {3/8 \over z-2i} } \,dz\\ &= 2\pi i\qty{ {1\over 4} + {3\over 8} + {3\over 8}} .\end{align*}$

exercise (Residues using partial fractions/principal parts):

Find all residues of the following function by writing it as a sum of principal parts at its poles: $\begin{align*} f(z) = {z^3 \over z^2 + 1} .\end{align*}$

#complex/exercise/completed

solution:

Use polynomial long division to write $\begin{align*} z^3 = z(z^2+1) - z \implies {z^3 \over z^2 + 1} = z - {z\over z^2 + 1} .\end{align*}$ Factor the latter part: $\begin{align*} {z\over z^2 + 1} = {a\over z+i} + {b\over z-i} \implies a(z-i) + b(z+i) = z ,\end{align*}$ evaluate at $z=i$ to get $b=1/2$ , and at $z=-i$ to get $a=1/2$ . Thus $\begin{align*} f(z) = z - {1/2 \over z+i} - {1/2 \over z-i} = P_\infty + P_{-i} + P_{i} ,\end{align*}$ yielding poles at $\pm i$ with residues $\begin{align*} \mathop{\mathrm{Res}}_{z=\infty} f(z) &= -1 \\ \mathop{\mathrm{Res}}_{z = i} f(z) &= -1/2 \\ \mathop{\mathrm{Res}}_{z = -i} f(z) &= -1/2 \\ .\end{align*}$

exercise (Residue from Laurent expansion: $1/(z - \sin(z))$):

Use a direct Laurent expansion to show $\begin{align*} \mathop{\mathrm{Res}}_{z=0} {1\over z-\sin(z)} = {3! \over 5\cdot 4} .\end{align*}$

Note the necessity: one doesn’t know the order of the pole at zero, so it’s unclear how many derivatives to take.

#complex/exercise/completed

solution:

Expand: $\begin{align*} {1\over z - \sin(z)} &= z^{-1}\qty{1 - z^{-1}\sin(z) }^{-1}\\ &= z^{-1}\qty{1 - z^{-1}\qty{ z - {1\over 3!}z^3 + {1\over 5!} z^5 - \cdots}}^{-1}\\ &= z^{-1}\qty{1 - \qty{ 1 - {1\over 3!}z^2 + {1\over 5!} z^4 - \cdots}}^{-1}\\ &= z^{-1}\qty{{1\over 3!}z^2 - {1\over 5!}z^4 + \cdots}^{-1}\\ &= z^{-1}\cdot 3! z^{-2} \qty{1 - {1\over 5!/3!}z^2 + \cdots}^{-1}\\ &= {3!\over z^3} \qty{1 \over 1 - \qty{ {1\over 5\cdot 4}z^2 + \cdots}} \\ &= {3!\over z^3}\qty{1 + \qty{{1\over 5\cdot 4}z^2} + \qty{{1\over 5\cdot 4}z^2}^2 + \cdots} \\ &= 3! z^{-3} + {3!\over 5\cdot 4}z^{-1}+ O(z) \\ .\end{align*}$

exercise (Computing residues: $1/z^2\sin(z)$):

Compute $\begin{align*} \mathop{\mathrm{Res}}_{z=0} {1\over z^2 \sin(z)} .\end{align*}$

#complex/exercise/completed

solution:

First expand $(\sin(z))^{-1}$ : $\begin{align*} {1\over \sin(z)} &= \qty{z - {1\over 3!}z^3 + {1\over 5!}z^5 -\cdots }^{-1}\\ &= z^{-1}\qty{1 - {1\over 3!}z^2 + {1\over 5!}z^4 - \cdots }^{-1}\\ &= z^{-1}\qty{1 + \qty{{1\over 3!}z^2 - {1\over 5!} z^4 + \cdots} + \qty{{1\over 3!}z^2 - \cdots}^2 + \cdots } \\ &= z^{-1}\qty{1 + {1\over 3!}z^2 \pm O(z^4) } ,\end{align*}$ using that $(1-x)^{-1}= 1 + x + x^2 + \cdots$ .

Thus $\begin{align*} z^{-2}\qty{\sin(z)}^{-1} &= z^{-2} \cdot z^{-1}\qty{1 + {1\over 3!}z^2 \pm O(z^4) } \\ &= z^{-3} + {1\over 3!}z^{-1}+ O(z) .\end{align*}$

Applying the formulas

exercise (Residue of $1/z^2 + 1$):

Use the rational function formula to compute the residues at $z=\pm i$ of $\begin{align*} f(z) \coloneqq{1\over z^2 + 1} .\end{align*}$

#complex/exercise/completed

solution:

Applying the rational function formula: $\begin{align*} \mathop{\mathrm{Res}}_{z=z_0}{1\over 1+z^2} &= {1\over 2z}\Big|_{z= z_0} \implies\\ \mathop{\mathrm{Res}}_{z=i}f(z) &= {1\over 2i} = -{i\over 2} \\ \mathop{\mathrm{Res}}_{z=-i}f(z) &= -{1\over 2i} = {i\over 2} .\end{align*}$

exercise (Residue of $1/z^n+1$):

Find the residue at $\omega_n \coloneqq e^{\pi i \over n}$ of $\begin{align*} f(z) = {1\over z^n + 1} .\end{align*}$

#complex/exercise/completed

solution (?):

Check that ${\frac{\partial }{\partial z}\,} z^n+1 = nz^{n-1}\neq 0$ for $z\neq 0$ , so this has no repeated roots since $z=0$ is not a root. Thus all of the poles are simple, so apply the rational function formula: $\begin{align*} \mathop{\mathrm{Res}}_{z=\zeta_{m}} {1\over z^n + 1} &= {1 \over nz^{n-1}}\Big|_{z=\omega_n} \\ &= {1\over n\omega_n^{n-1}} \\ &= {\omega_n^{1-n}\over n} \\ &= -{\omega_n \over n} ,\end{align*}$ which follows from expanding $\begin{align*} \omega_n^{1-n} = e^{i\pi (1-n) \over n} = e^{i\pi\over n}e^{-i\pi n \over n} = e^{i\pi \over n}\cdot (-1) = -\omega_n .\end{align*}$ .

exercise (Residues at $\infty$):

Compute $\begin{align*} &\mathop{\mathrm{Res}}_{z=\infty}e^z\\ &\mathop{\mathrm{Res}}_{z=\infty}{z-1\over z+1} .\end{align*}$

#complex/exercise/completed

solution:

In parts:

For $e^z$ :
- Integral formula: $\mathop{\mathrm{Res}}_{z=\infty}f(z) = -{1\over 2\pi }\int_\gamma f(z)\,dz$ where $\gamma$ encloses all singularities of $f$ , but $e^z$ is entire, so this integral is zero and thus the residue is zero.
- Inversion formula: expand $z^{-2}f(1/z)$ about $z=0$ to obtain $\begin{align*} {1\over z^2}e^{1\over z} = z^{-2}\sum_{k\geq 0}z^{-k}/k! = \sum_{k\geq 0}z^{-k-2}/k! = z^{-2} + z^{-3} + {1\over 2!}z^{-4} + { \mathsf{O}} (z^{-5}) ,\end{align*}$ so the residue is zero.
For ${z+1\over z-1}$ :
- Integral formula: $\begin{align*} \mathop{\mathrm{Res}}_{z=\infty} &= -{1\over 2\pi}\int_{{\left\lvert {z} \right\rvert} = 2} {z-1\over z+1}\,dz\\ &= - \mathop{\mathrm{Res}}_{z=-1} {z-1\over z+1} \\ &= - (-2) \\ &= 2 .\end{align*}$
- Inversion formula: $\begin{align*} {1\over z^2}{ z^{-1}- 1 \over z^{-1}+ 1} &= z^{-2}{1 - z \over 1 + z} \\ &= z^{-2}(z-1)\sum_{k\geq 0} (-z)^k \\ &= z^{-2} + 2z^{-1}-2 + 2z - { \mathsf{O}} (z^2) ,\end{align*}$ which has residue 2.